
&DifferentialD u &DifferentialD t = 1 − b + 1 u + a u 2 ν, d ν dt = b u − a u 2 ν , The dynamics of this system are given by: The brusselator is a mathematical model for chemical oscillation. x t is the population of the prey at time t, y t is the population of the predator at time t, 0 ĭes ≔ &DifferentialD &DifferentialD t x t = &alpha x t − b x t y t, &DifferentialD &DifferentialD t y t = − &beta y t + c x t y t : &DifferentialD &DifferentialD t x t = α x t − b x t y t, &DifferentialD &DifferentialD t y t = − β y t + c x t y t , 2.5, 0, 0.2, 2, 0, 2, 0.2, axes = box, linecolor = blue, dirfield = 600, arrows = fish, color = magnitude ĭes := &DifferentialD &DifferentialD t x t = − &beta 1 + &beta 2 x t, &DifferentialD &DifferentialD t y t = &beta 2 x t − &beta 3 y t : &DifferentialD x_1 &DifferentialD t = − β 1 + β 2 x 1, &DifferentialD x_2 &DifferentialD t = β 2 x 1 − β 3 x 2. We obtain the following system of two first order differential equations representing these relations. In addition, cells die from compartment A with the rate β 1 x 1 and die from compartment B with the rate β 3 x 2. Cells pass from compartment A to B with a rate of β 2 x 1.
Let x 1 be the number of arrested cells (sitting on the boundary of the tissue in compartment A) and x 2 be the number of cells that have invaded the target tissue (compartment B). This is a two-compartment model for the metastasis of cancer, that is, the spread of cancer cells through the boundary of an organ tissue. &DifferentialD v &DifferentialD t + μ v + ω 2 x = 0, v = &DifferentialD x &DifferentialD tĭes := &DifferentialD &DifferentialD t x t = v t, &DifferentialD &DifferentialD t v t = − &mu v t − &omega 2 x t :ĭEtools :- DEplot des, x t, v t, t = 0. One can convert this linear second order differential equation into a system of two first order differential equations by letting v = &DifferentialD x &DifferentialD t, that is, v is the velocity, obtaining: The term μ &DifferentialD x &DifferentialD t models the friction with the table where μ is the damping coefficient. Here ω = k m, k is the constant of spring, m is the mass. &DifferentialD 2 x &DifferentialD t 2 + μ &DifferentialD x &DifferentialD t + &omega 2 x = 0

Assuming it is proportional to the velocity of the mass, we obtain the following equation
When the mass slides over the table there will be a frictional force applied to the mass in the opposite direction of motion. − k x = m &DifferentialD 2 x &DifferentialD t 2 Since f = m a, that is, force equals mass times acceleration, we have the following second order differential equation which governs the motion of the mass. Hooke's law states that the force exerted by the spring on the mass is − k x where k is the spring constant, that is, the force is proportional to the displacement x of the mass from the rest position. If the mass is moved so that the spring is stretched or compressed, a force is applied to the mass. Let x = 0 be the point where the mass is in rest position.

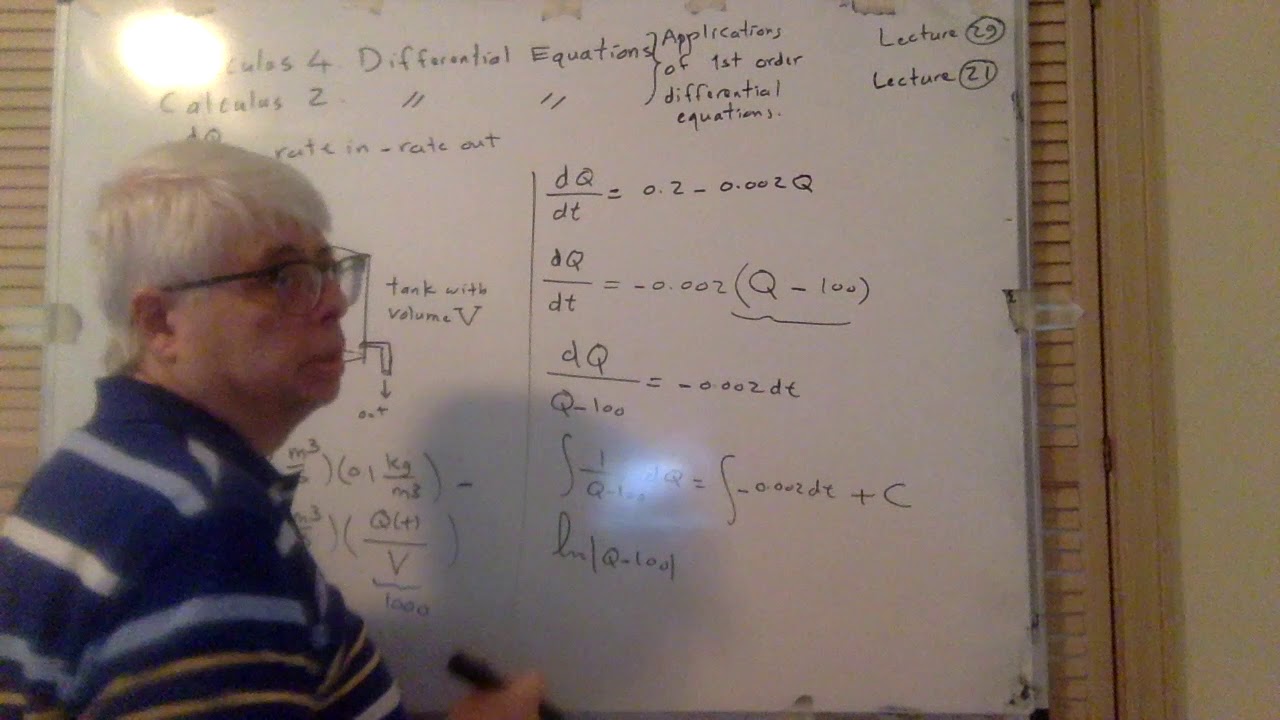
Suppose a mass m, which is attached to a spring which is fixed to a wall, is resting on a table. Foremost is how we can develop a differential equation by thinking about the “total rate = rate in - rate out” model.This example page describes the default models (available on startup) for the DEplot differential system tool.


 0 kommentar(er)
0 kommentar(er)
